You are here: Home > British Military > Ordnance
Whitworth Guns > Experiments
Mr. Joseph Whitworth produces a large display of guns and projectiles prepared on his system, which differs in many points from that of his great competitor and rival, Sir William Armstrong. Whitworth for a considerable time rejected “built-up” guns, and formed all natures of his ordnance, however heavy, of solid masses of what is called “homogeneous metal,” a puzzle-the-vulgar phrase, for tough steely iron, without fibre, i.e., with minute saccaroid crystallization. Latterly, however, he has employed for his larger guns in many instances this material in heavy thicknesses, for an internal tube, reinforced at the breech-end with one or more plies of rings, of the same, or of other qualities of iron, shrunk on with initial tension; and in this we are quite sure he is right.
Whitworth’s guns are most of them muzzle-loaders; and he has been apparently only forced into devising his own peculiar form of breech-loader, by the ignorant and interested clamour that has been raised in favour of breech-loading, insisting upon imaginary values and virtues which it does not possess; indeed, all its real virtues and applicabilities might be summed up in a few sentences. This much we feel bound to say, that if breech-loading must be employed, Whitworth’s arrangement is, to our views, greatly to be preferred, as it leaves the breech part of the gun (the vital point) much less reduced in strength, than does that of Sir William Armstrong. For large heavy guns, however, the one arrangement becomes just as unworkable as the other.
Whitworth’s cannon are bored cylindrical, and then rifled to a hexagonal form – the sides of the figure not quite joining, but being connected by narrow strips of the cylinder left between them. Thus both the bore of the gun and the mid section of the shot are twelve-sided, the duodecagon having alternate long and very short sides. The twist is uniform, and for all natures varies from one in twenty, to one in twenty-two calibres. It is the most rapid twist that has been employed by any rifle artillerist. Mr. Whitworth attaches great importance to this extreme suddenness of twist, and consequent high velocity of rotation of his shot in flight. We cannot avoid thinking, however, that he has carried this velocity of rotation far beyond what is at all necessary to insure as great accuracy in flight (and especially in the heavy natures of projectiles) as is important, or that can be supported, by the other conditions unavoidable in practical gunnery.
Our conclusion is not shaken by the fact that we cannot find that Mr. Whitworth (or indeed any one else as yet) has established on fundamental principles a necessary velocity of rotation, for a given projectile, with given initial velocity, such, that it shall within a given range, maintain a given ratio of deviative inaccuracy; and until this be done, the ipse dixit that an enormously high velocity, or any particular velocity of rotation, is indispensable, is mere empiricism.
If Whitworth’s velocity of rotation be excessive, for procuring all practically useful accuracy, then is he sacrificing to it, two of the highest points of merit, of his guns and their practice – viz, wasting the strength of his gun on rotation, which might otherwise be devoted to resisting a larger charge, so as to produce a still greater initial velocity of shot.
Fig. 637
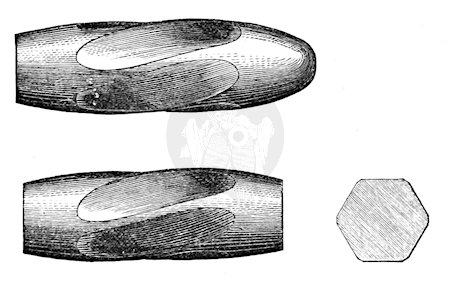
Fig. 638 and Fig. 639
Whitworth Shot
Whitworth’s projectiles are of two forms and two materials. For ordinary service, both shot and shell are elongated parabolic spindles, curtate in the rere by a plane transverse to the axis (Fig. 637 and 638), and are made of cast-iron. For penetrative purposes, against armour plates in particular, he employs a parabolic spindle of homogeneous iron or of tempered steel, curtate at both ends (Fig. 639), the forward or striking end being slightly convex in late examples. The length varies from three to five calibres. The shot fit the guns with extremely little windage – as little, in fact, as will enable them to be rammed home in the muzzle-loaders; and in the case of the smaller natures of breech-loaders, we believe, even less. The shot are cast in dry sand, moulded by very simple but effective tools, and are afterwards tripped over in the lathe, and the six spiral flancs planed upon them by peculiarly adapted machines.
The first form of shot approaches much more nearly to what Piobert has shown to be the form of least aerial resistance, than that of any other before the world. The result, of having produced an extremely strong gun, competent to bear the explosion of a comparatively heavy charge with a very elongated and therefore relatively heavy projectile, and by construction and exactness of workmanship, having so exactly adapted his gun and his projectile together, that there is practically almost no windage, while the whole force of the explosion is absorbed in producing the rotation and flight of the shot, and none absorbed in its deformation – is, that Whitworth may justly boast of having conferred the highest velocity upon a large projectile ever yet given by man. Thus it is that he has realized an initial velocity in his smaller natures of shot of very nearly 2000 feet per second. The following will serve to compare this with the velocities of other ordnance:
Table III.—INITIAL VELOCITIES
| At 45° elevation, 36-inch shells, | 500 to 600 feet per second. |
| At 45° elevation, 13-inch shells, | 800 feet per second. |
| Hollow shot (level), 10 inch gun, | 1200 to 1300 feet per sec. |
| Hollow shot (level), 8-inch gun, | 1400 to 1500 feet per sec. |
| Solid shot (level), 68-pounder, | 1600 feet nearly per sec. |
| Solid shot (level), 32-pounder, | 1690 feet nearly per sec. |
| Whitworth, 70-pounder, | 1260 feet nearly per sec. |
| Whitworth, 12-pounder, | 1905 feet nearly per sec. |
All these are round numbers. We believe the velocity of the Armstrong shot from the breech-loaders has not exceeded from 1100 to 1200 feet per second. With the shunt gun there is no reason why he should not equal that of Whitworth.
The penetrative power of any shot against a nearly rigid body, such as an armour plate, being proportionate (neglecting some small quantities) to
M V2 / D
M being the mass, V the velocity of impact, and D the calibre of the projectile; while the resisting power of the armour plate (again neglecting small quantities) is only proportionate to
M x D V
M being the mass cut or broken out, D its diameter, and V the velocity with which it is cut or fractured out: it is obvious how enormous is the advantage that Whitworth obtains by this high velocity and small diameter of projectiles.